AS066/KH0079 - Indexed Pendant Sums

Drawings:

Overview	Detail View
All Khipus' Ascher Sum Fieldmarks	Right-Handed XRay
All Indexed Pendant Sum Relationships	Left-Handed XRay
This Khipu's Fieldmark Catalog	Right-Handed Sum Detail
This Khipu's Drawing	Left-Handed Sum Detail

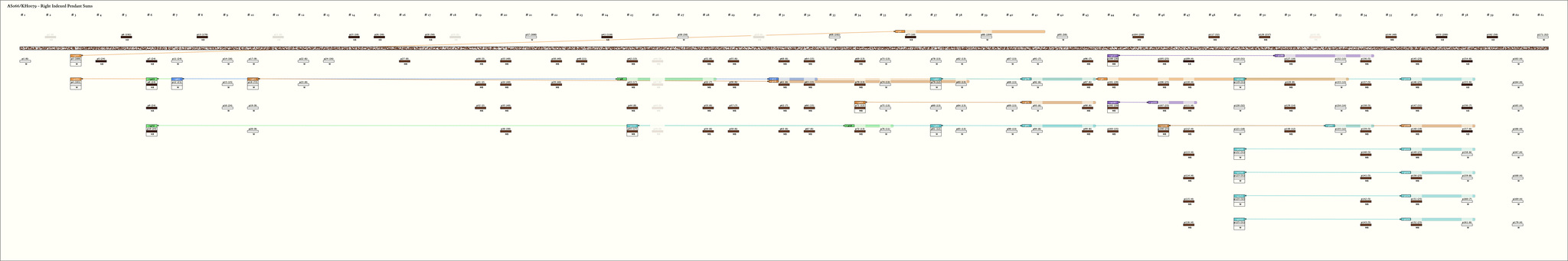
Right Handed Sums: # Sums = 18, Max # Summands = 6, (Min, Mean, Max) Sum Values = (12, 38, 160)
Click on Image to View Larger

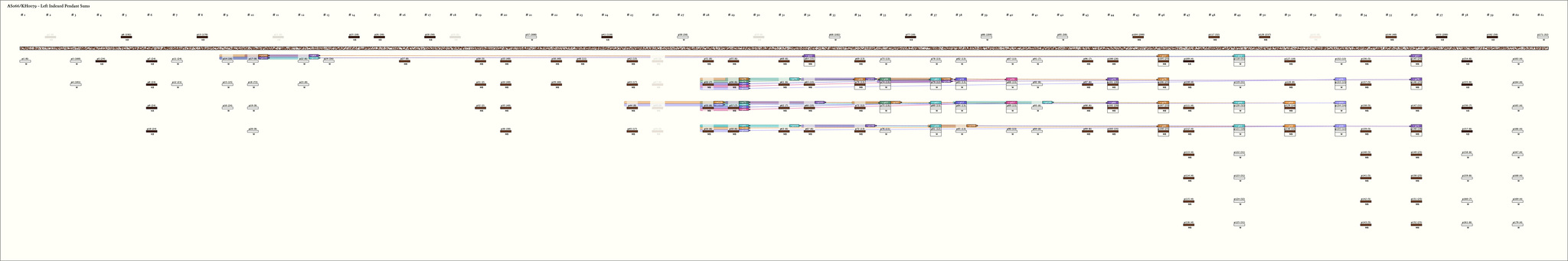
Left Handed Sums: # Sums = 28, Max # Summands = 3, (Min, Mean, Max) Sum Values = (12, 18, 32)
Click on Image to View Larger

Right Handed Sum Detail: - Click on column name to sort

#	Sum Cord	Sum Cord Value	# Summands	Summands
1	p3_{3, 1} : 160_W	160	6	p77: 10_KB + p78: 13_W + p82: 13_W + p86: 104_W + p87: 13_W + p91: 7_W
2	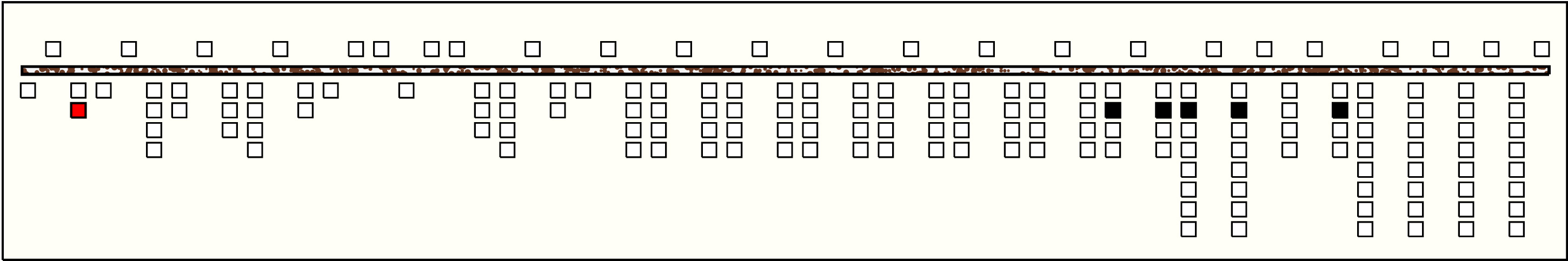p4_{3, 2} : 101_W	101	5	p101: 28_MB + p106: 25_MB + p110: 4_MB + p119: 31_W + p133: 13_W
3	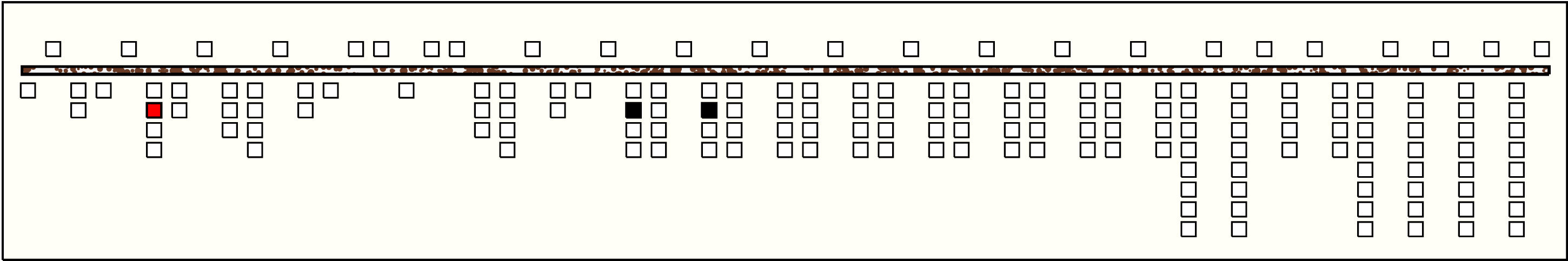p8_{6, 2} : 24_KB	24	2	p43: 17_MB + p52: 7_MB
4	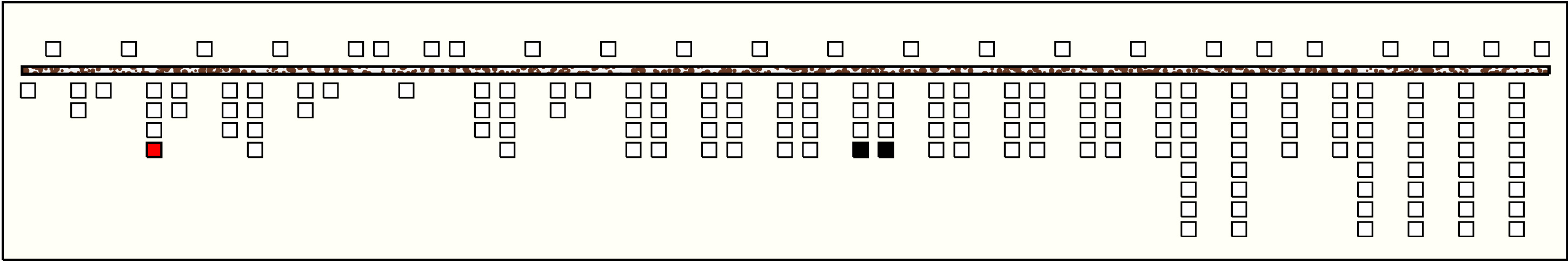p10_{6, 4} : 24_KB	24	2	p72: 13_MB + p76: 11_W
5	p12_{7, 2} : 21_W	21	2	p61: 6_MB + p65: 15_MB
6	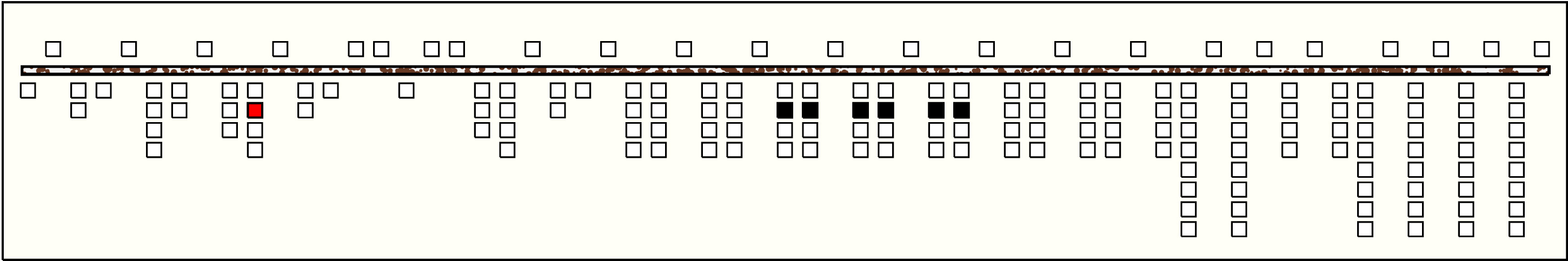p18_{10, 2} : 72_W	72	6	p61: 6_MB + p65: 15_MB + p70: 13_MB + p74: 13_W + p79: 12_W + p83: 13_W
7	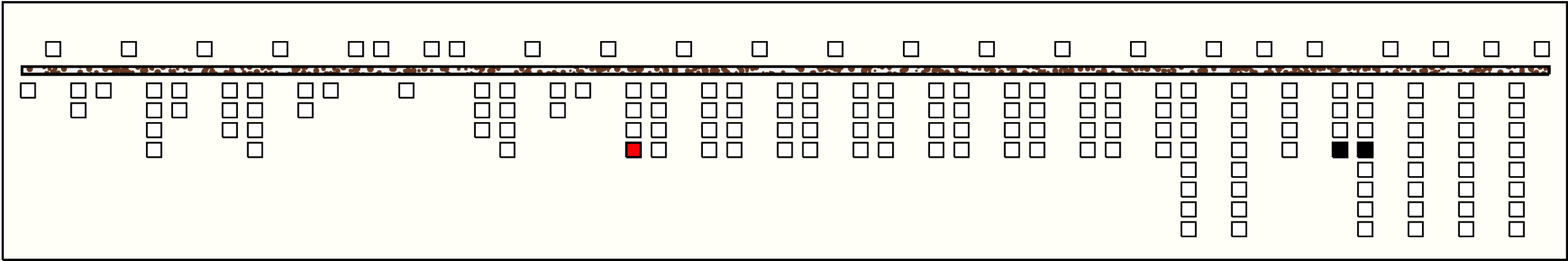p45_{25, 4} : 17_MB	17	2	p135: 12_W + p139: 5_MB
8	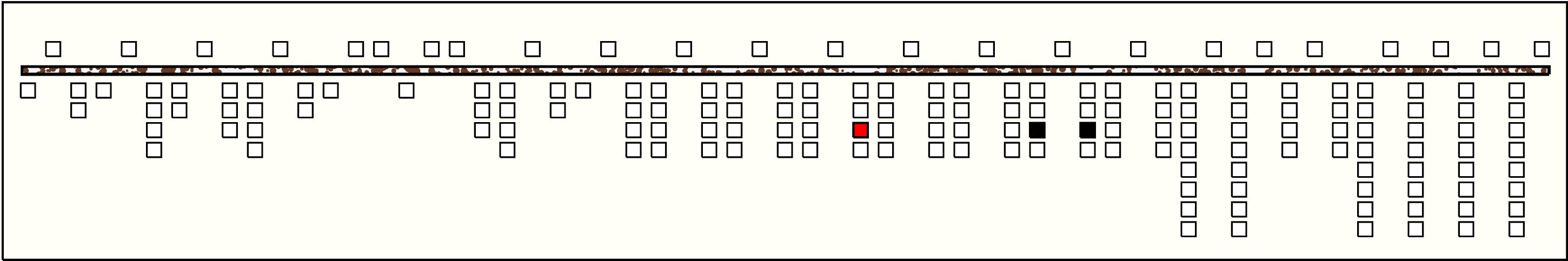p71_{34, 3} : 12_MB	12	2	p93: 6_W + p98: 6_MB
9	p79_{37, 2} : 12_W	12	2	p92: 6_W + p97: 6_MB
10	p81_{37, 4} : 12_W	12	2	p94: 6_W + p99: 6_MB
11	p100_{44, 1} : 28_MB	28	3	p127: 10_MB + p132: 13_W + p136: 5_MB
12	p102_{44, 3} : 29_MB	29	2	p107: 25_MB + p111: 4_MB
13	p108_{46, 4} : 25_MB	25	2	p148: 19_MB + p157: 6_KB
14	p119_{49, 2} : 31_W	31	2	p146: 25_MB + p155: 6_KB
15	p122_{49, 5} : 31_W	31	2	p149: 25_MB + p158: 6_W
16	p123_{49, 6} : 31_W	31	2	p150: 25_MB + p159: 6_W
17	p124_{49, 7} : 32_W	32	2	p151: 25_MB + p160: 7_W
18	p125_{49, 8} : 31_W	31	2	p152: 25_MB + p161: 6_W

Left Handed Sum Detail: - Click on column name to sort

#	Sum Cord	Sum Cord Value	# Summands	Summands
1	p64_{32, 1} : 15_MB	15	2	p17: 9_W + p22: 6_W
2	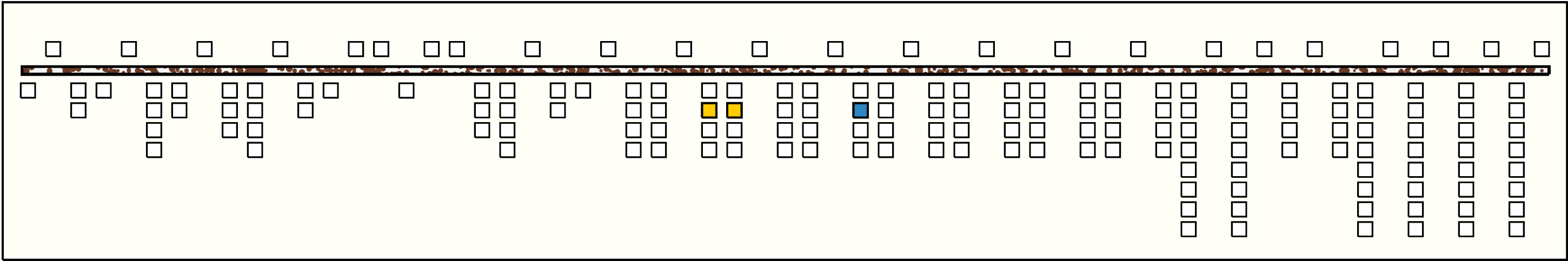p70_{34, 2} : 13_MB	13	2	p52: 7_MB + p56: 6_MB
3	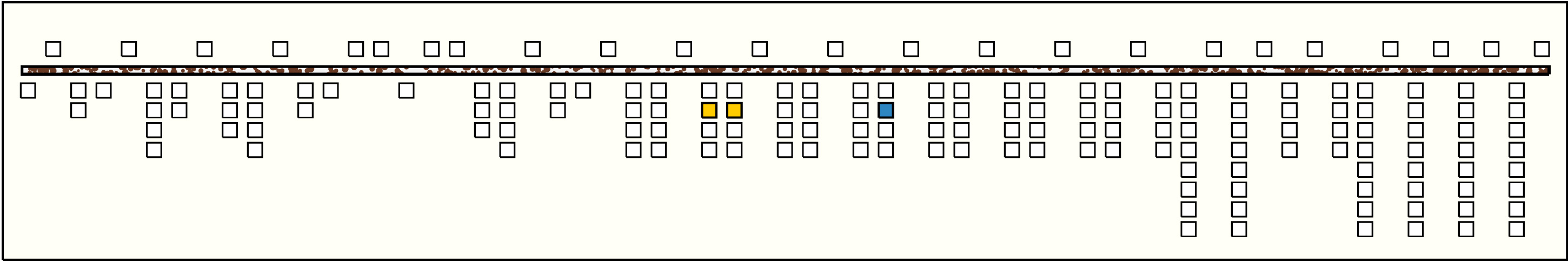p74_{35, 2} : 13_W	13	2	p52: 7_MB + p56: 6_MB
4	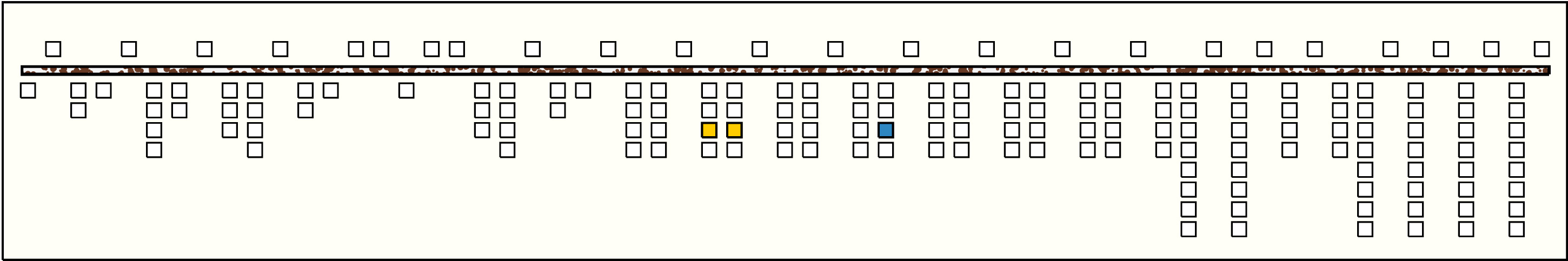p75_{35, 3} : 13_W	13	2	p53: 6_MB + p57: 7_MB
5	p79_{37, 2} : 12_W	12	2	p56: 6_MB + p61: 6_MB
6	p80_{37, 3} : 13_W	13	2	p53: 6_MB + p57: 7_MB
7	p81_{37, 4} : 12_W	12	2	p54: 6_MB + p58: 6_MB
8	p83_{38, 2} : 13_W	13	2	p52: 7_MB + p56: 6_MB
9	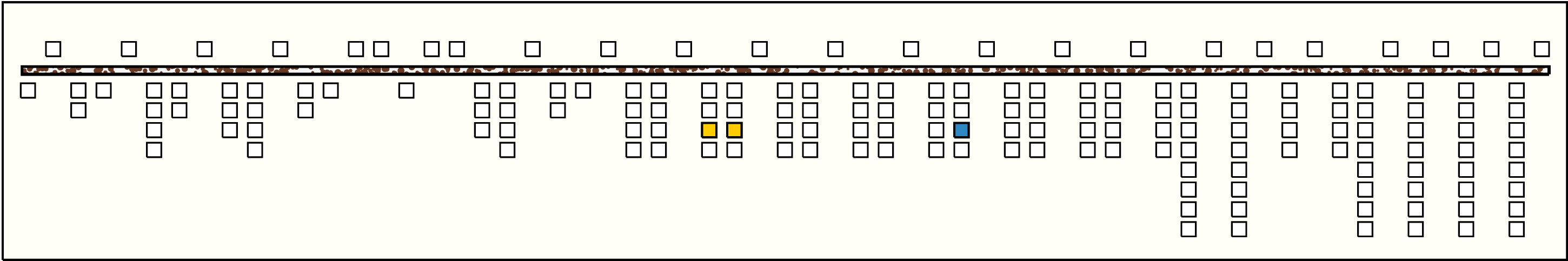p84_{38, 3} : 13_W	13	2	p53: 6_MB + p57: 7_MB
10	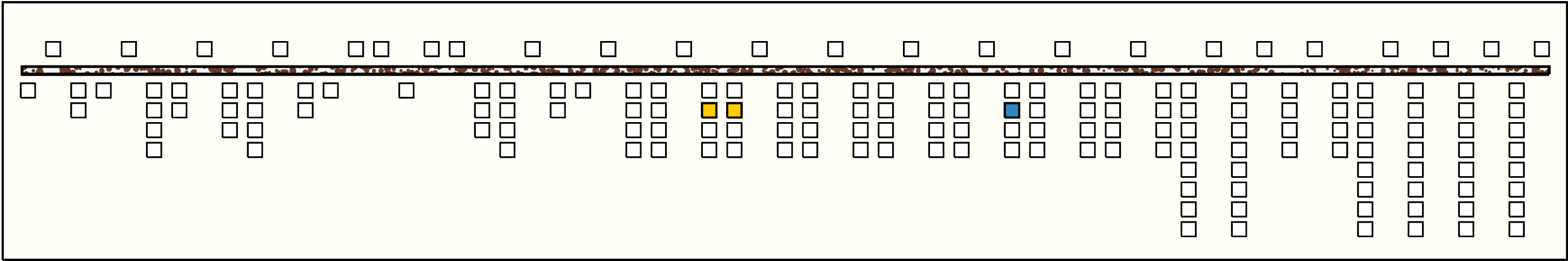p88_{40, 2} : 13_W	13	2	p52: 7_MB + p56: 6_MB
11	p89_{40, 3} : 13_W	13	2	p53: 6_MB + p57: 7_MB
12	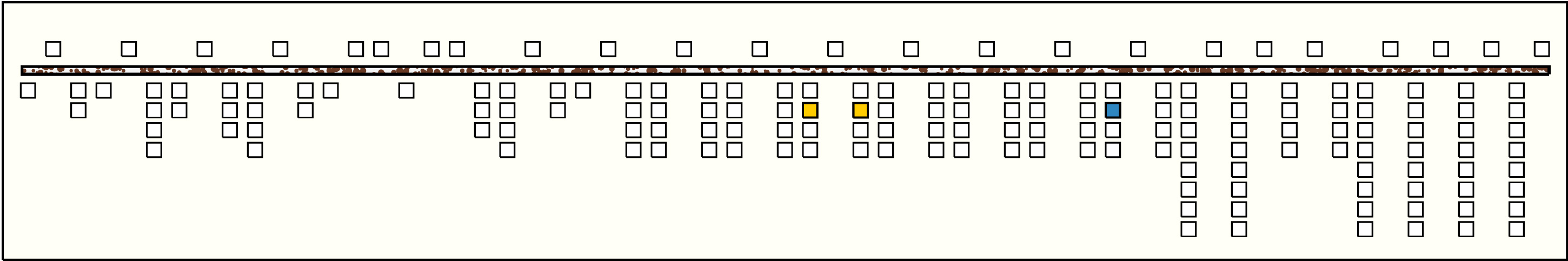p101_{44, 2} : 28_MB	28	2	p65: 15_MB + p70: 13_MB
13	p102_{44, 3} : 29_MB	29	3	p57: 7_MB + p62: 7_MB + p66: 15_MB
14	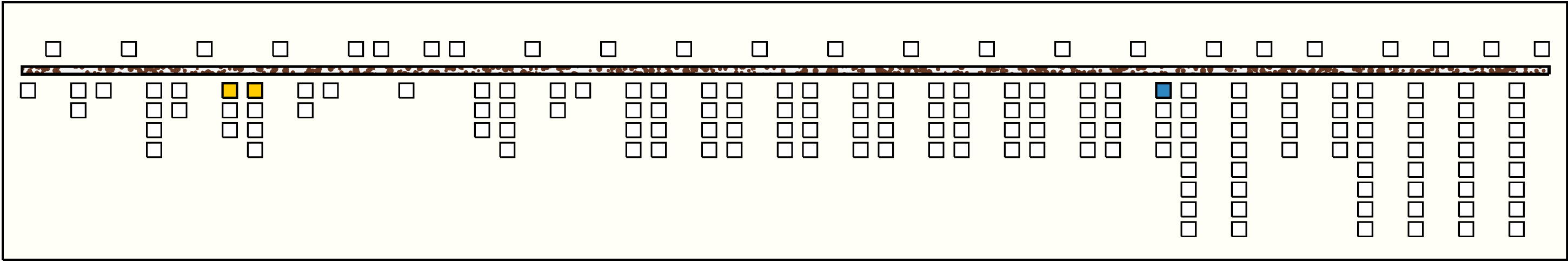p105_{46, 1} : 25_MB	25	2	p14: 16_W + p17: 9_W
15	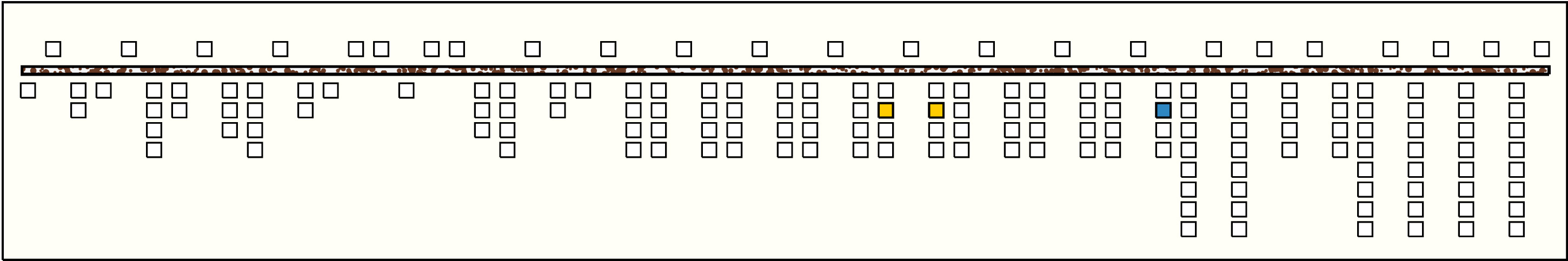p106_{46, 2} : 25_MB	25	2	p74: 13_W + p79: 12_W
16	p107_{46, 3} : 25_MB	25	2	p71: 12_MB + p75: 13_W
17	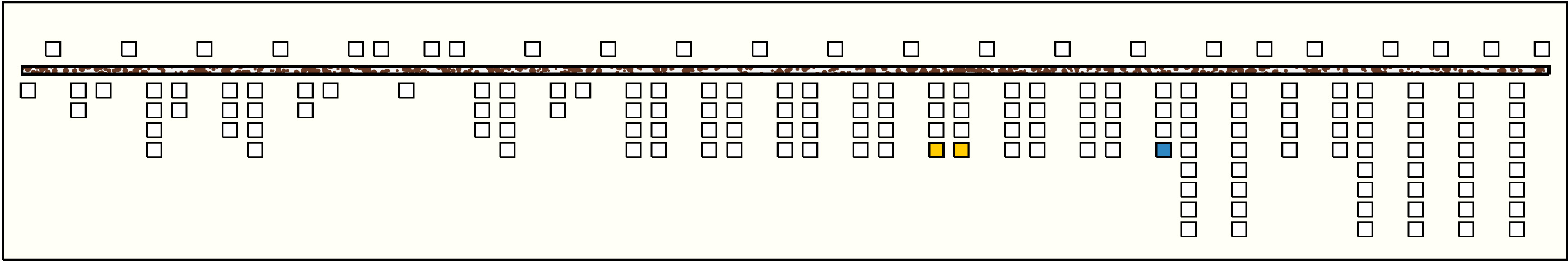p108_{46, 4} : 25_MB	25	2	p81: 12_W + p85: 13_W
18	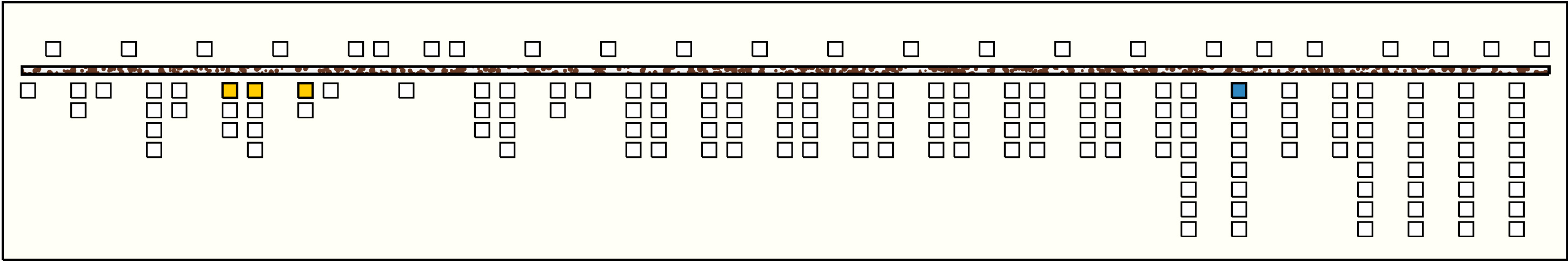p118_{49, 1} : 31_W	31	3	p14: 16_W + p17: 9_W + p22: 6_W
19	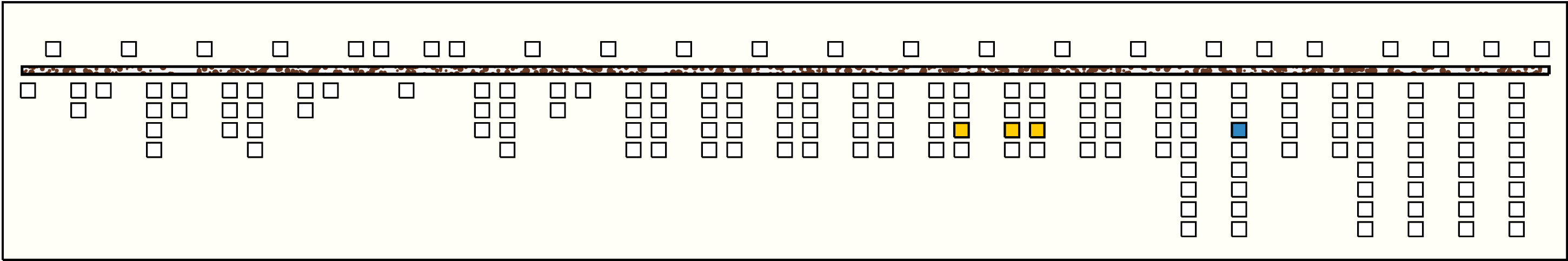p120_{49, 3} : 32_W	32	3	p84: 13_W + p89: 13_W + p93: 6_W
20	p121_{49, 4} : 18_W	18	3	p54: 6_MB + p58: 6_MB + p63: 6_MB
21	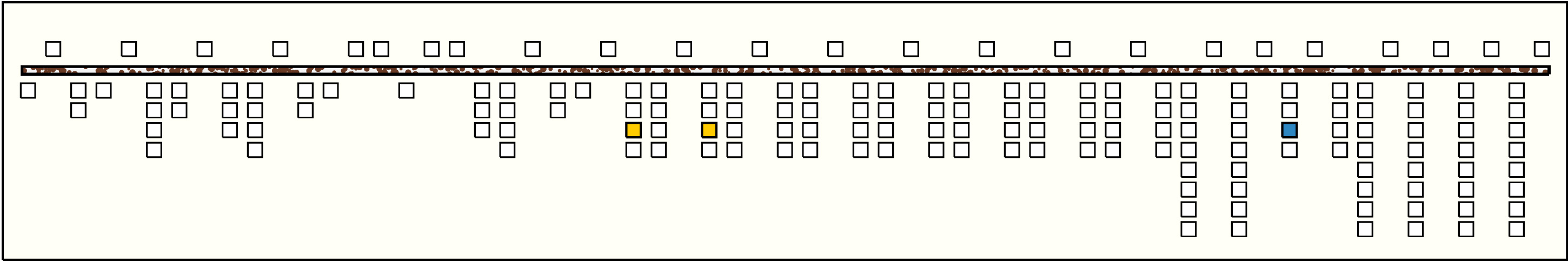p129_{51, 3} : 14_MB	14	2	p44: 8_MB + p53: 6_MB
22	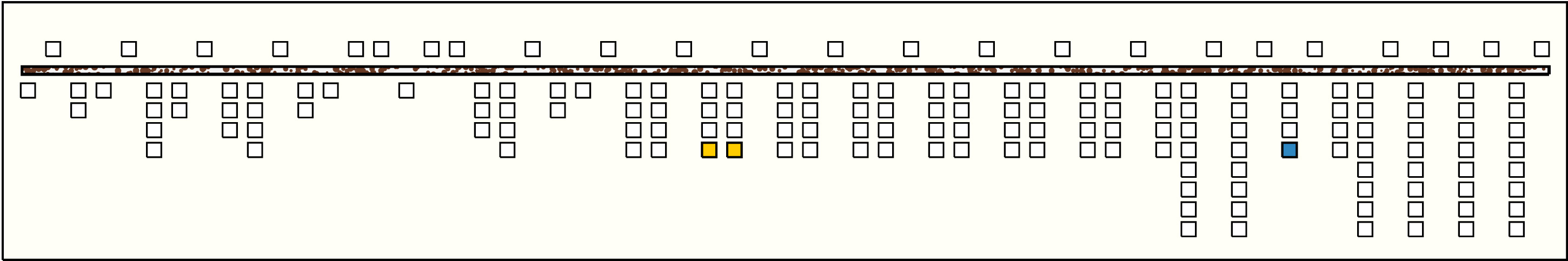p130_{51, 4} : 12_MB	12	2	p54: 6_MB + p58: 6_MB
23	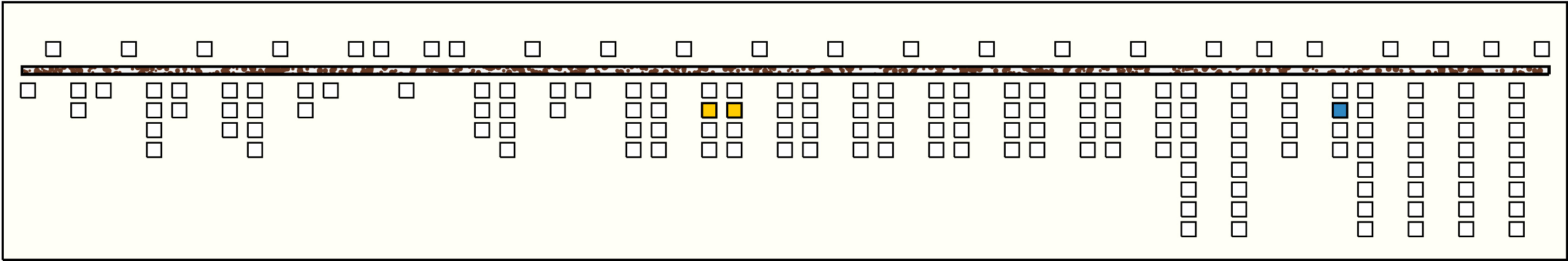p133_{53, 2} : 13_W	13	2	p52: 7_MB + p56: 6_MB
24	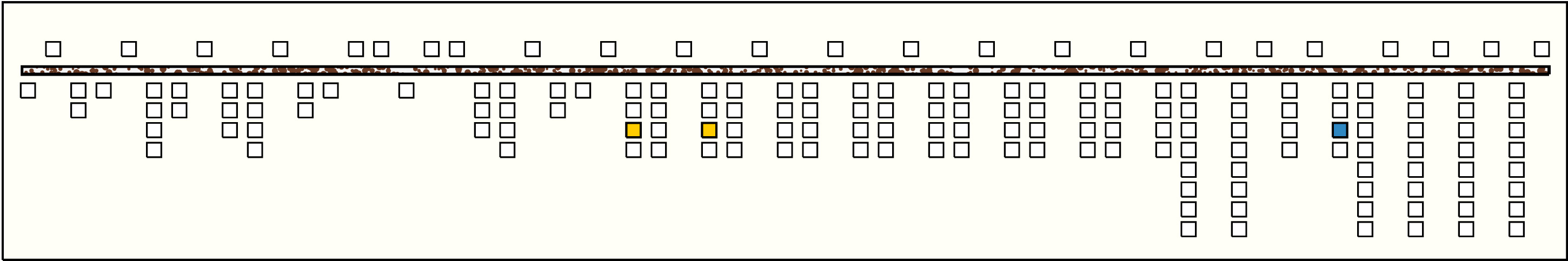p134_{53, 3} : 14_W	14	2	p44: 8_MB + p53: 6_MB
25	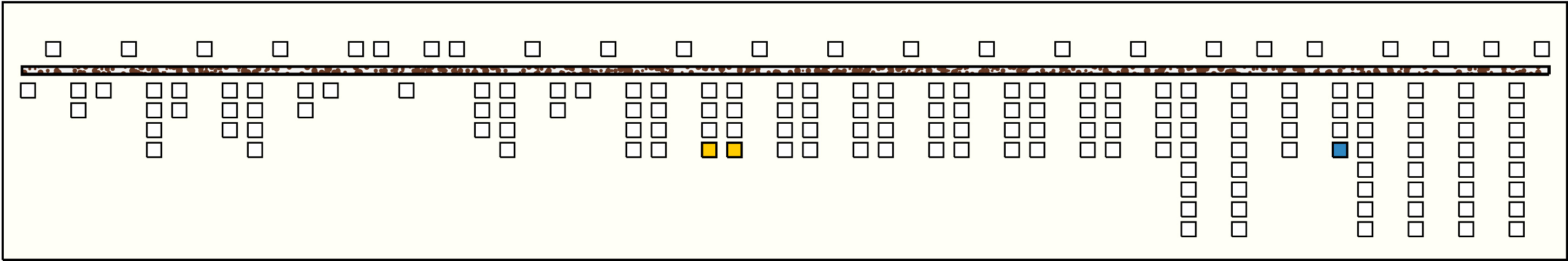p135_{53, 4} : 12_W	12	2	p54: 6_MB + p58: 6_MB
26	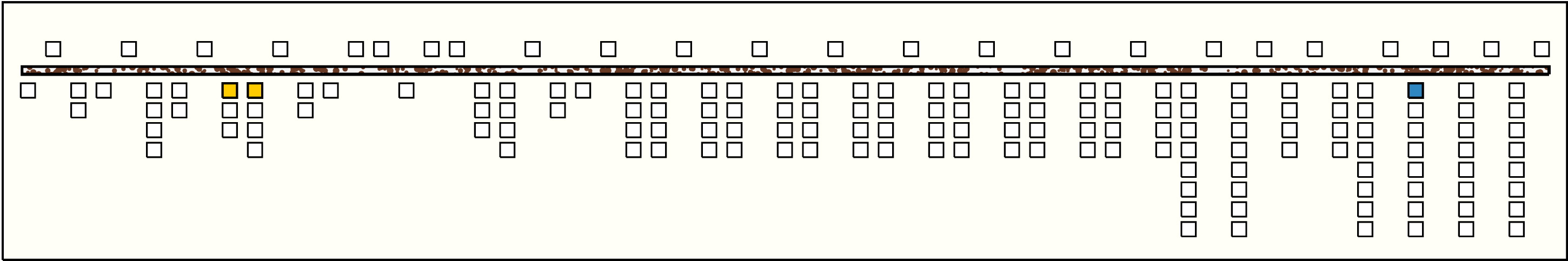p145_{56, 1} : 25_MB	25	2	p14: 16_W + p17: 9_W
27	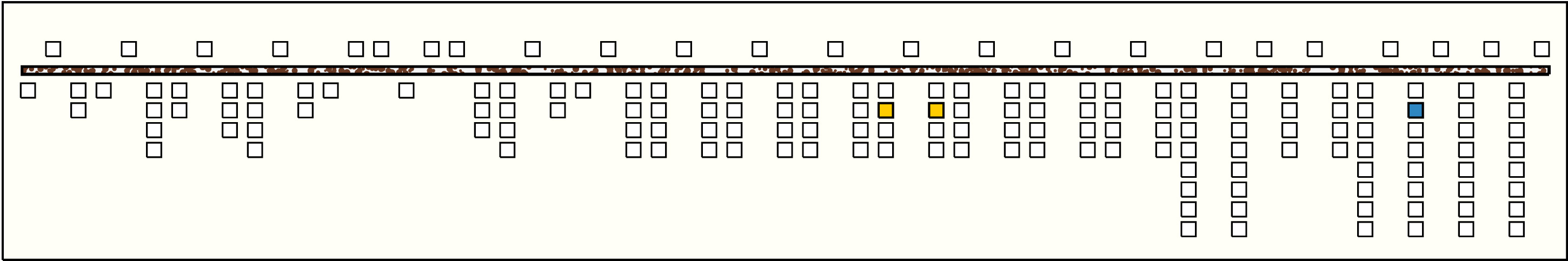p146_{56, 2} : 25_MB	25	2	p74: 13_W + p79: 12_W
28	p148_{56, 4} : 19_MB	19	2	p67: 6_MB + p72: 13_MB

Khipu Notes:

Ascher Databook Notes:

This khipu is associated with AS059-KH0080. See discussion under AS059.
From the discoloration of the main cord, and by extrapolation based on the spacing of the complete groups, we hypothesize that this khipu contained 24 groups of 8 pendants.
Each of the groups had a top cord. Of the original 80 pendants of the first 10 groups, only 35 remain and so the first complete group starts with pendant 36. Of the last 14 groups, no pendants are missing.
Two loose broken cords were associated with this khipu: one, colored DB, had a value of 50; the other, colored AB, had a value of 14.
Both common forms of top cord attachment are present on this khipu. In groups 18, 19, and 21-24, the top cord unites the pendants. In the remaining groups, the top cord is attached to the main cord in the center of the group.
The first pendant of the 18th group (P92) is a different color than the rest of the group reinforcing the idea that this is a place where a change occurs.
All pendant cords within a group are the same color with the exception of group 18 noted above and groups 16, 20, and 23 in which the first 4 pendants are one color and the last 4 another color.
In 2 of these groups the top cord is the same color as the first 4 pendants in the group. The third group has the top cord missing so its color is unknown.
Only 6 groups have the top cord the same color as all the pendants in the group. Five of these groups are the only groups for which all pendants in the group have the same value.
The color of the top cords for groups 8-11 (BB, W, DB, W) are repeated for the next 4 groups (12-15).
With the exception of group 19, all groups that have all pendant values and top cord value present show the following relationship:
\[ \sum\limits_{j=1}^4 P_{ij} = \sum\limits_{j=5}^8 P_{ij} = \frac{top\_value}{2}\;\;\;for\;(i=13,15,16,17,18,21,22,23,24) \]
Where one of the three parts of the relationship is unknown due to breakage, the other two still appear:
\[ \sum\limits_{j=1}^4 P_{ij} = \sum\limits_{j=5}^8 P_{ij} = \frac{top\_value}{2}\;\;\;for\;(i=12,14,17) \]

\[ \sum\limits_{j=1}^4 P_{ij} = \frac{top\_value}{2}\;\;\;for\;(i=9) \]

\[ \sum\limits_{j=5}^8 P_{ij} = \frac{top\_value}{2}\;\;\;for\;(i=2,10,11) \]

(Note: P_ij is the value of the j^th pendant in the i^th group.)
As noted in observation 6, five groups have all equal pendant values. The sum value on the top cord must, therefore, be a multiple of 8.
In some cases where the top value is not a multiple of 8, the pendant values can be viewed as top value/ 8 rounded to the nearest integers.
This is seen in groups 11,12,16,23 where the sums are 50. The pendant values are 6x6 + 2x7.
Similarly in group 9, the pendant values can be viewed as rounded to the nearest multiple of 10. That is 150 = 3x40 + 1x30.
Groups 17 and 19 both have the same relationship among the first 4 pendants:
\[ P_{1} = P_{2} = \frac{x}{4} + 3 \]

\[ P_{3} = \frac{x}{4} + 4 \]

\[ P_{4} = \frac{x}{4} - 10 \]
And as a result:
\[ P_{1}+P_{2} = \frac{x}{2} + 6,\;\;\;\;\;\;\;P_{3}+P_{4} = \frac{x}{2} - 6\]
For group 17, where X=100 and for group 19, where X=112.
Since group 19 is the only group for which P1 + P2 + P3 + P4 ≠ P5 + P6 + P7 + P8, it is interesting to note that also:
\[ P_{1}+P_{2}+P_{3}+P_{4} = \frac{top\_value}{2}-6\;\;\;where\;\frac{top\_value}{2}\;is\;rounded\;DOWN \]

\[ P_{5}+P_{6}+P_{7}+P_{8} = \frac{top\_value}{2}+6\;\;\;where\;\frac{top\_value}{2}\;is\;rounded\;UP \]