AS041/KH0052 - Pendant Pendant Sums

Drawings:

Overview	Detail View
All Khipus' Ascher Sum Fieldmarks	Right-Handed XRay
All Pendant Pendant Sum Relationships	Left-Handed XRay
This Khipu's Fieldmark Catalog	Right-Handed Sum Detail
This Khipu's Drawing	Left-Handed Sum Detail

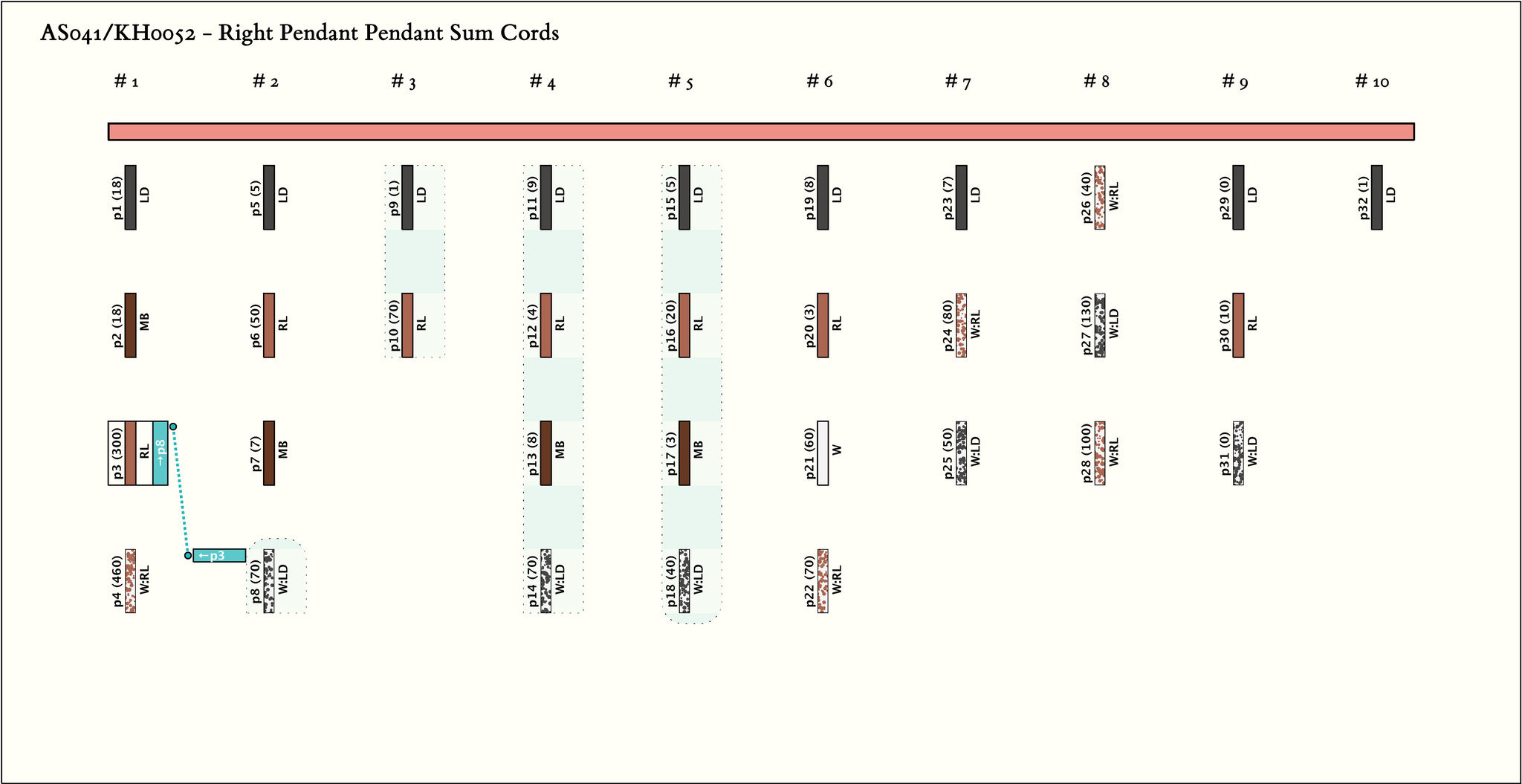
Right Handed Sums:     # Sums = 1,  Max # Summands = 11,   (Min, Mean, Max) Sum Values = (300, 300, 300)
Click on Image to View Larger

Left Handed Sums:     # Sums = 2,  Max # Summands = 3,   (Min, Mean, Max) Sum Values = (80, 105, 130)
Click on Image to View Larger

Right Handed Sum Detail: - Click on column name to sort

#	Color	Sum Schema	Sum Cord	Sum Cord Value	# Summands	Summands
1		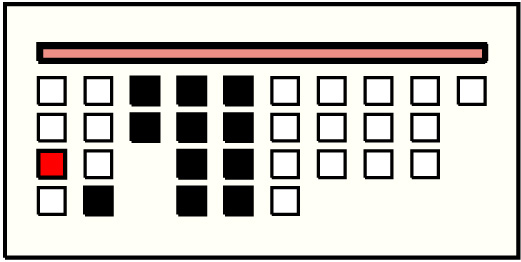	p31, 3 : 300RL	300	11	p8: 70W:LD + p9: 1LD + p10: 70RL + p11: 9LD + p12: 4RL + p13: 8MB + p14: 70W:LD + p15: 5LD + p16: 20RL + p17: 3MB + p18: 40W:LD

Left Handed Sum Detail: - Click on column name to sort

#	Color	Sum Schema	Sum Cord	Sum Cord Value	# Summands	Summands
1		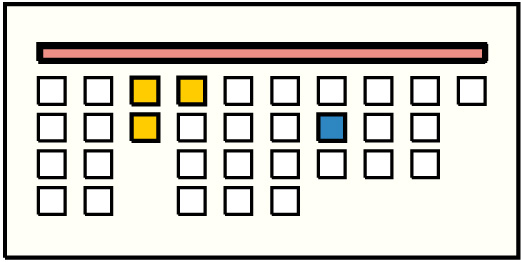	p247, 2 : 80W:RL	80	3	p9: 1LD + p10: 70RL + p11: 9LD
2			p278, 2 : 130W:LD	130	2	p24: 80W:RL + p25: 50W:LD

Khipu Notes:

Ascher Databook Notes:

1. By spacing there are 10 groups of 4, 4,2, 4, 4, 4,3, 3,3, 1 pendants. There is some consistency of color pattern. LD appears in 9 of the 10 groups in position 1 and in no other positions.
   Groups 2, 4, and 5 have the same color pattern: LD, RL, MD, LD:W.
   Group 1 is similar, but with the colors of positions 2 and 3 reversed and RL:W instead of LD: W.
   The association of position 3 in Group 2 with position 2 in other groups, and of RL:W with LD:W, is further seen when examining numerical relationships.
2. The values in Group 1 are the sums o f the values in the subsequent groups. Where pendants of the same color are in similar positions in different groups, their values are related. However, the sum seems to be more related to pendant color than position. While other sum statements might be as valid, one which accounts for most values follows. (Note: P_ij is the value of the j^th pendant in the i^thth group. P_ijsk is the value of the k^th subsidiary on the j^th pendant in the i^th group.)
   
   \[P_{11}+P_{11s1}+P_{11s2}=P_{21}+P_{31}+P_{41}+P_{51}+P_{61}+P_{71}\;for\;all\;LD \]
   \[P_{12}=P_{23}+P_{43}+P_{53}\;for\;all\;(MB) \]
   \[P_{12s1}=P_{43s1}\;for\;all\;(W) \]
   \[P_{13s1}=P_{52}+P_{22s1}+P_{24s1}+P_{44s1}\;for\;all\;(RL) \]
   
   
   
   \[^{(RL)}{P_{13}=P_{22}+P_{32}+P_{92}}\;+\;^{(W)}{P_{63}}\;+\;^{(LD:W)}{P_{24}+P_{54}}\;for\;all\;(LD:W)\]
   \[P_{14s1}=P_{32s1}\;for\;all\;(LD:W)\]
   \[^{(LD:W)}{P_{14}}=^{(LD:W)}{P_{22}}\;\;+^{(LD-W)}{P_{73}}\;\;+^{(RL:W)}{P_{64}+P_{72}+P_{72s1}+P_{81}+P_{83}}\;for\;all\;(LD:W)\]
   
   
   Inexact sums on subsidiaries of position 1 in Group 1:
   \[P_{11s1s1}+P_{11s2s2}\;exceeds\;P_{61s1}+P_{71s1}\;by\;6\;for\;all\;MB \]
   \[P_{11s1s2}\;exceeds\;P_{31s1}\;by\;1\;for\;all\;W \]
   \[P_{11s2s1}\;exceeds\;P_{21s1}+P_{51s1}+P_{71s1}\;by\;2\;for\;all\;RL \]
   
   
   Seven values in groups 2-10 unaccounted for in sums :
   \[^{(LD:W)}{P_{14}}=\;^{(LD:W)}{P_{22}}\;+\;^{(LD-W)}{P_{73}}\;+\;^{(RL:W)}{P_{64}+P_{72}+P_{72s1}+P_{81}+P_{83}}\;for\;all\;(LD:W)\]