AS211/KH0231 - Pendant Pendant Sums

Drawings:

Overview	Detail View
All Khipus' Ascher Sum Fieldmarks	Right-Handed XRay
All Pendant Pendant Sum Relationships	Left-Handed XRay
This Khipu's Fieldmark Catalog	Right-Handed Sum Detail
This Khipu's Drawing	Left-Handed Sum Detail

Right Handed Sums: # Sums = 3, Max # Summands = 8, (Min, Mean, Max) Sum Values = (23, 31, 37)
Click on Image to View Larger

Left Handed Sums: # Sums = 3, Max # Summands = 8, (Min, Mean, Max) Sum Values = (13, 21, 34)
Click on Image to View Larger

Right Handed Sum Detail: - Click on column name to sort

#	Sum Cord	Sum Cord Value	# Summands	Summands
1	p10_{1, 10} : 23_B	23	3	p34: 5_B + p35: 2_B + p36: 16_W
2	p19_{2, 1} : 34_W	34	6	p39: 5_W + p40: 6_W + p41: 5_W + p42: 5_W + p43: 0_W + p44: 13_B
3	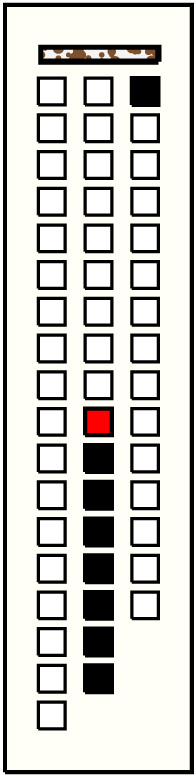p28_{2, 10} : 37_B	37	8	p29: 2_B + p30: 2_B + p31: 3_B + p32: 5_B + p33: 2_B + p34: 5_B + p35: 2_B + p36: 16_W

Left Handed Sum Detail: - Click on column name to sort

#	Sum Cord	Sum Cord Value	# Summands	Summands
1	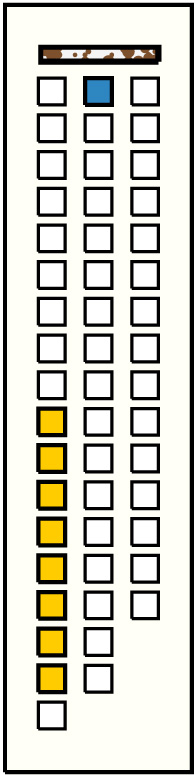p19_{2, 1} : 34_W	34	8	p10: 23_B + p11: 0_B + p12: 2_B + p13: 1_B + p14: 0_B + p15: 5_B + p16: 2_B + p17: 1_B
2	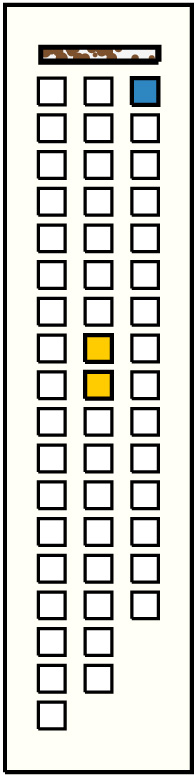p36_{3, 1} : 16_W	16	2	p26: 9_W + p27: 7_W
3	p44_{3, 9} : 13_B	13	2	p23: 6_W + p24: 7_W

Khipu Notes:

Ascher Databook Notes:

A cord fragment was tied to the main cord just before P19. The cord color is B and it is broken at both ends. Beginning at the broken end nearest the single knots there is a space of 4.5, 3s, space of 8.5, 3L, space of 13.5, broken end. It is unlikely that the fragment is part of broken P50, P10s1, or P18s1 because the knots would not be aligned with the others in the group. The fragment's value (33) would make it unlikely to be part of broken P48 or the pendant that may be missing just after P35. These would be expected to have values less than 10. When the fragment was tied to the main cord is unknown but it does not appear to have been done recently.
A discoloration on the main cord suggests that another pendant was once attached just after P35. We assume this in the observations that follow.
By spacing the khipu is separated into three groups of pendants. By color the groups are each divided into two subgroups. The first two groups have 9 pendants per subgroup and the third group has subgroups of 8 and 7 pendants. Therefore the khipu can be described as:

P_ijk where
i=(1,2,3) (group by space)
j=(1,2) (subgroup by color)
k=(1,...,9) for i=(1,2) (position in subgroup)
k=(1,...,8) for i=3, j=1 (position in subgroup)
k=(1,...,7) for i=3, j=2 (position in subgroup)
Each pendant in group 1 has a subsidiary. Each subsidiary is the color of its host pendant. All subsidiary values are less than 10. No subsidiaries appear in groups 2 and 3.
Each group consists of a subgroup of W cords followed by a subgroup of B cords. That is:

For all i,k:
- P_i1k is W
- P_i2k is B
The first pendant in each subgroup contains the maximum value in the subgroup. Depending on the group, the values are in the teens, twenties, or thirties. All other pendant values are less than 10. Specifically:

For j=(1,2)
- P_1j1 = 22 & 1
- 34 ≤ P_2j1 ≤ 37
- 13 ≤ P_3j1 ≤ 14 & 2
- 9 ≥ P_ijk ≥ 0 for i=(1,2,3); j=(1,2); k≠(1)
With the exception of the first position (and the unknown value due to breakage) the values in the first subgroup of group 2, position by position, are greater han or equal to those in the second subgroup. Also, position by position, they are greater than or equal to those in each subgroup in group 1. That is:

For j=(2,...,8)
- P_21j ≥ P_22j
For j=(1,...,9)
- P_21j ≥ P_11j
- P_21j ≥ P_12j
Several values are repeated in the corresponding positions of the subgroups of group 1. They are:

For j=(1,2)
- P_1j1 = 22 & 1
- P_1j2 = 0 with subsidiary va1ue 0
- P_1j4 = 1
- P_1j5 = 0 with subsidiary va1ue 0
- P_1j7 = 2 with subsidiary va1ue 0