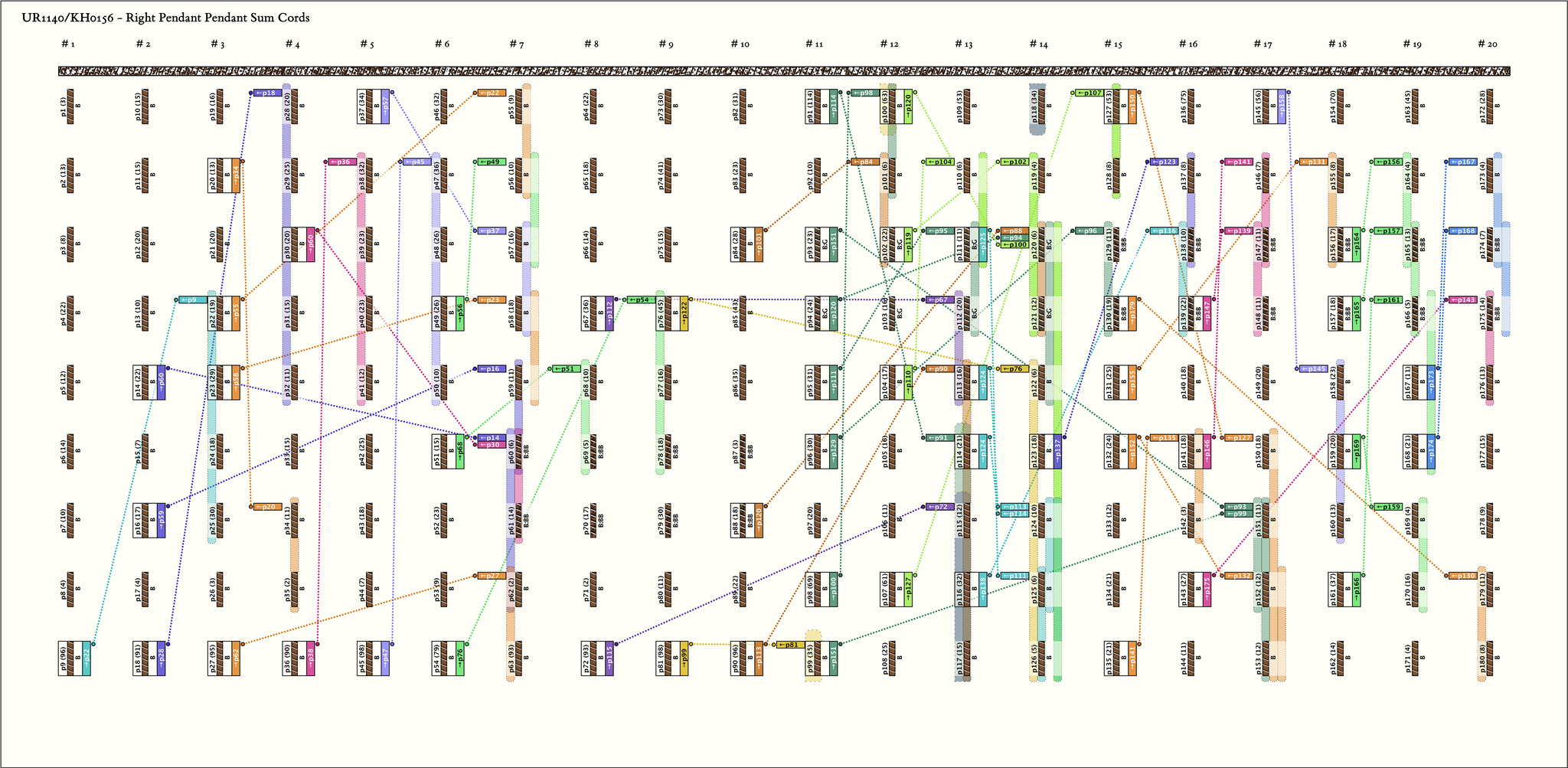
UR1140/KH0156 - Pendant Pendant Sums

Drawings:

Overview	Detail View
All Khipus' Ascher Sum Fieldmarks	Right-Handed XRay
All Pendant Pendant Sum Relationships	Left-Handed XRay
This Khipu's Fieldmark Catalog	Right-Handed Sum Detail
This Khipu's Drawing	Left-Handed Sum Detail

Right Handed Sums: # Sums = 53, Max # Summands = 7, (Min, Mean, Max) Sum Values = (11, 40, 114)
Click on Image to View Larger

Left Handed Sums: # Sums = 49, Max # Summands = 6, (Min, Mean, Max) Sum Values = (11, 40, 114)
Click on Image to View Larger

Right Handed Sum Detail: - Click on column name to sort

#	Sum Cord	Sum Cord Value	# Summands	Summands
1	p9_{1, 9} : 96_B	96	4	p22: 19_B + p23: 29_B + p24: 18_B + p25: 30_B
2	p14_{2, 5} : 22_B	22	3	p60: 6_B:BB + p61: 14_B:BB + p62: 2_B
3	p16_{2, 7} : 17_B	17	2	p59: 11_B + p60: 6_B:BB
4	p18_{2, 9} : 91_B	91	5	p28: 20_B + p29: 25_B + p30: 20_B + p31: 15_B + p32: 11_B
5	p20_{3, 2} : 13_B	13	2	p34: 11_B + p35: 2_B
6	p22_{3, 4} : 19_B	19	2	p55: 9_B + p56: 10_B
7	p23_{3, 5} : 29_B	29	2	p58: 18_B + p59: 11_B
8	p27_{3, 9} : 95_B	95	2	p62: 2_B + p63: 93_B
9	p30_{4, 3} : 20_B	20	2	p60: 6_B:BB + p61: 14_B:BB
10	p36_{4, 9} : 90_B	90	4	p38: 32_B + p39: 23_B + p40: 23_B + p41: 12_B
11	p37_{5, 1} : 34_B	34	2	p57: 16_B + p58: 18_B
12	p45_{5, 9} : 98_B	98	4	p47: 36_B + p48: 26_B + p49: 26_B + p50: 10_B
13	p49_{6, 4} : 26_B	26	2	p56: 10_B + p57: 16_B
14	p51_{6, 6} : 15_B	15	2	p68: 10_B + p69: 5_B:BB
15	p54_{6, 9} : 79_B	79	3	p76: 45_B + p77: 16_B + p78: 18_B:BB
16	p67_{8, 4} : 36_B	36	2	p112: 20_B:G + p113: 16_B
17	p72_{8, 9} : 93_B	93	4	p115: 12_B + p116: 32_B + p117: 15_B + p118: 34_B
18	p76_{9, 4} : 45_B	45	5	p122: 6_B + p123: 18_B + p124: 10_B + p125: 6_B + p126: 5_B
19	p81_{9, 9} : 98_B	98	2	p99: 35_B + p100: 63_B
20	p84_{10, 3} : 28_B	28	2	p101: 6_B + p102: 22_B:G
21	p88_{10, 7} : 18_B:BB	18	2	p120: 6_B:G + p121: 12_B:G
22	p90_{10, 9} : 96_B	96	5	p113: 16_B + p114: 21_B + p115: 12_B + p116: 32_B + p117: 15_B
23	p91_{11, 1} : 114_B	114	5	p114: 21_B + p115: 12_B + p116: 32_B + p117: 15_B + p118: 34_B
24	p93_{11, 3} : 23_B:G	23	2	p151: 11_B + p152: 12_B
25	p94_{11, 4} : 24_B:G	24	3	p120: 6_B:G + p121: 12_B:G + p122: 6_B
26	p95_{11, 5} : 31_B	31	2	p111: 11_B:G + p112: 20_B:G
27	p96_{11, 6} : 30_B	30	2	p129: 11_B:BB + p130: 19_B:BB
28	p98_{11, 8} : 69_B	69	2	p100: 63_B + p101: 6_B
29	p99_{11, 9} : 35_B	35	3	p151: 11_B + p152: 12_B + p153: 12_B
30	p100_{12, 1} : 63_B	63	7	p120: 6_B:G + p121: 12_B:G + p122: 6_B + p123: 18_B + p124: 10_B + p125: 6_B + p126: 5_B
31	p102_{12, 3} : 22_B:G	22	3	p119: 4_B + p120: 6_B:G + p121: 12_B:G
32	p104_{12, 5} : 17_B	17	2	p110: 6_B + p111: 11_B:G
33	p107_{12, 8} : 61_B	61	2	p127: 53_B + p128: 8_B
34	p111_{13, 3} : 11_B:G	11	2	p125: 6_B + p126: 5_B
35	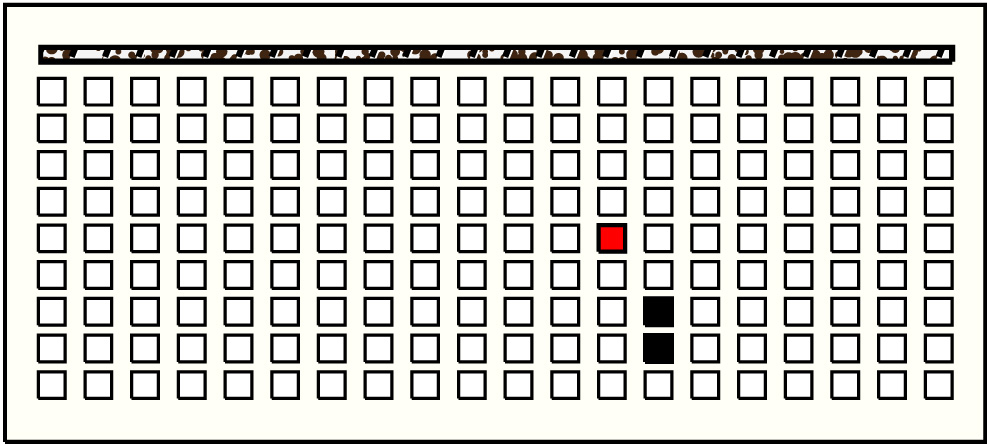p113_{13, 5} : 16_B	16	2	p124: 10_B + p125: 6_B
36	p114_{13, 6} : 21_B	21	3	p124: 10_B + p125: 6_B + p126: 5_B
37	p116_{13, 8} : 32_B	32	2	p138: 10_B:BB + p139: 22_B:BB
38	p123_{14, 6} : 18_B	18	2	p137: 8_B + p138: 10_B:BB
39	p127_{15, 1} : 53_B	53	4	p150: 18_B + p151: 11_B + p152: 12_B + p153: 12_B
40	p130_{15, 4} : 19_B:BB	19	2	p179: 11_B + p180: 8_B
41	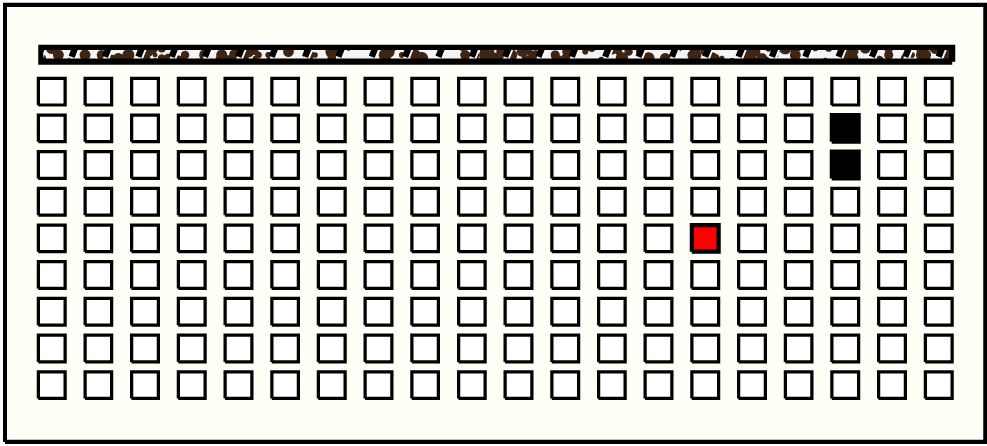p131_{15, 5} : 25_B	25	2	p155: 8_B + p156: 17_B:BB
42	p132_{15, 6} : 24_B	24	2	p152: 12_B + p153: 12_B
43	p135_{15, 9} : 21_B	21	2	p141: 18_B + p142: 3_B
44	p139_{16, 4} : 22_B:BB	22	2	p147: 11_B:BB + p148: 11_B:BB
45	p141_{16, 6} : 18_B	18	2	p146: 7_B + p147: 11_B:BB
46	p143_{16, 8} : 27_B	27	2	p175: 14_B:BB + p176: 13_B
47	p145_{17, 1} : 56_B	56	3	p158: 23_B + p159: 20_B + p160: 13_B
48	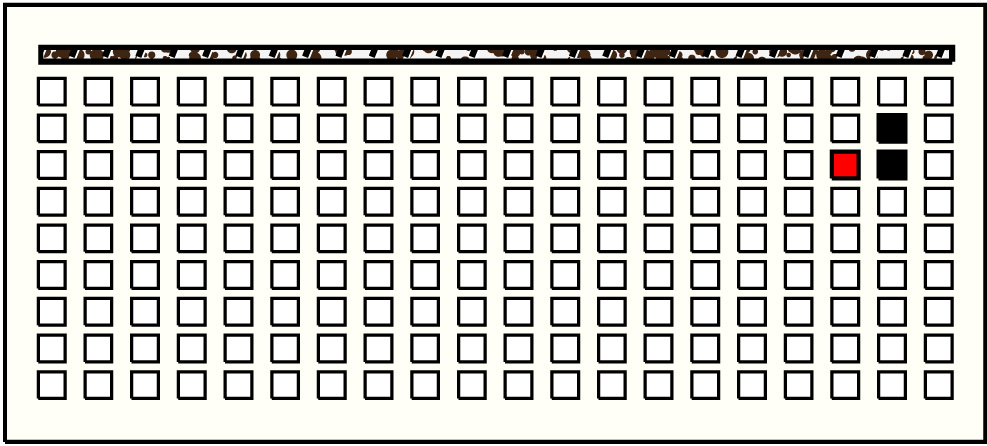p156_{18, 3} : 17_B:BB	17	2	p164: 4_B + p165: 13_B:BB
49	p157_{18, 4} : 18_B:BB	18	2	p165: 13_B:BB + p166: 5_B:BB
50	p159_{18, 6} : 20_B	20	2	p169: 4_B + p170: 16_B
51	p161_{18, 8} : 37_B	37	3	p166: 5_B:BB + p167: 11_B + p168: 21_B
52	p167_{19, 5} : 11_B	11	2	p173: 4_B + p174: 7_B:BB
53	p168_{19, 6} : 21_B	21	2	p174: 7_B:BB + p175: 14_B:BB

Left Handed Sum Detail: - Click on column name to sort

#	Sum Cord	Sum Cord Value	# Summands	Summands
1	p19_{3, 1} : 16_B	16	2	p1: 3_B + p2: 13_B
2	p25_{3, 7} : 30_B	30	2	p12: 20_B + p13: 10_B
3	p27_{3, 9} : 95_B	95	2	p17: 4_B + p18: 91_B
4	p37_{5, 1} : 34_B	34	2	p4: 22_B + p5: 12_B
5	p38_{5, 2} : 32_B	32	2	p13: 10_B + p14: 22_B
6	p45_{5, 9} : 98_B	98	2	p26: 3_B + p27: 95_B
7	p47_{6, 2} : 36_B	36	3	p5: 12_B + p6: 14_B + p7: 10_B
8	p48_{6, 3} : 26_B	26	2	p33: 15_B + p34: 11_B
9	p54_{6, 9} : 79_B	79	6	p2: 13_B + p3: 8_B + p4: 22_B + p5: 12_B + p6: 14_B + p7: 10_B
10	p61_{7, 7} : 14_B:BB	14	2	p7: 10_B + p8: 4_B
11	p64_{8, 1} : 22_B	22	3	p60: 6_B:BB + p61: 14_B:BB + p62: 2_B
12	p67_{8, 4} : 36_B	36	2	p49: 26_B + p50: 10_B
13	p70_{8, 7} : 17_B:BB	17	2	p59: 11_B + p60: 6_B:BB
14	p74_{9, 2} : 41_B	41	3	p31: 15_B + p32: 11_B + p33: 15_B
15	p75_{9, 3} : 15_B	15	2	p68: 10_B + p69: 5_B:BB
16	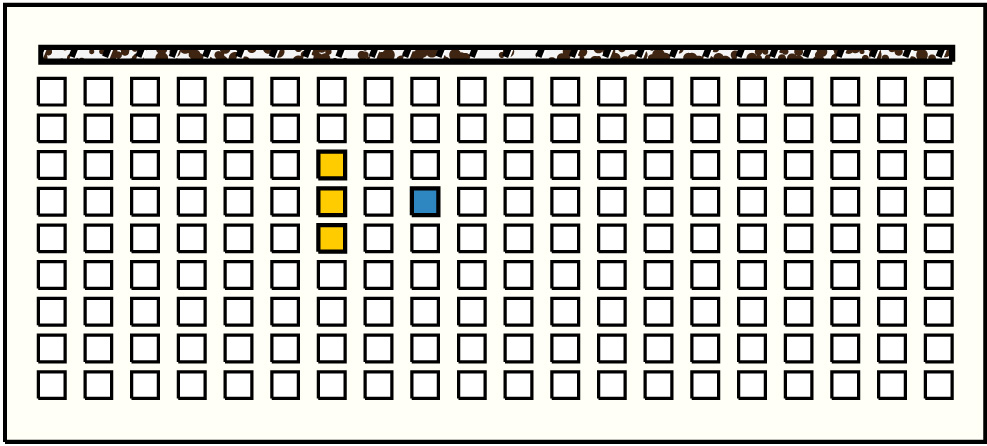p76_{9, 4} : 45_B	45	3	p57: 16_B + p58: 18_B + p59: 11_B
17	p77_{9, 5} : 16_B	16	2	p61: 14_B:BB + p62: 2_B
18	p81_{9, 9} : 98_B	98	3	p54: 79_B + p55: 9_B + p56: 10_B
19	p82_{10, 1} : 31_B	31	3	p59: 11_B + p60: 6_B:BB + p61: 14_B:BB
20	p84_{10, 3} : 28_B	28	3	p33: 15_B + p34: 11_B + p35: 2_B
21	p85_{10, 4} : 43_B	43	2	p42: 25_B + p43: 18_B
22	p86_{10, 5} : 35_B	35	3	p58: 18_B + p59: 11_B + p60: 6_B:BB
23	p89_{10, 8} : 22_B	22	2	p69: 5_B:BB + p70: 17_B:BB
24	p90_{10, 9} : 96_B	96	4	p22: 19_B + p23: 29_B + p24: 18_B + p25: 30_B
25	p91_{11, 1} : 114_B	114	4	p54: 79_B + p55: 9_B + p56: 10_B + p57: 16_B
26	p94_{11, 4} : 24_B:G	24	3	p69: 5_B:BB + p70: 17_B:BB + p71: 2_B
27	p97_{11, 7} : 20_B	20	2	p60: 6_B:BB + p61: 14_B:BB
28	p98_{11, 8} : 69_B	69	5	p2: 13_B + p3: 8_B + p4: 22_B + p5: 12_B + p6: 14_B
29	p108_{12, 9} : 25_B	25	2	p50: 10_B + p51: 15_B
30	p109_{13, 1} : 53_B	53	4	p55: 9_B + p56: 10_B + p57: 16_B + p58: 18_B
31	p114_{13, 6} : 21_B	21	2	p87: 3_B:BB + p88: 18_B:BB
32	p116_{13, 8} : 32_B	32	3	p68: 10_B + p69: 5_B:BB + p70: 17_B:BB
33	p118_{14, 1} : 34_B	34	2	p77: 16_B + p78: 18_B:BB
34	p127_{15, 1} : 53_B	53	3	p117: 15_B + p118: 34_B + p119: 4_B
35	p129_{15, 3} : 11_B:BB	11	2	p125: 6_B + p126: 5_B
36	p132_{15, 6} : 24_B	24	2	p122: 6_B + p123: 18_B
37	p134_{15, 8} : 21_B	21	3	p124: 10_B + p125: 6_B + p126: 5_B
38	p136_{16, 1} : 75_B	75	4	p77: 16_B + p78: 18_B:BB + p79: 30_B:BB + p80: 11_B
39	p139_{16, 4} : 22_B:BB	22	3	p119: 4_B + p120: 6_B:G + p121: 12_B:G
40	p143_{16, 8} : 27_B	27	2	p105: 16_B + p106: 11_B
41	p145_{17, 1} : 56_B	56	6	p119: 4_B + p120: 6_B:G + p121: 12_B:G + p122: 6_B + p123: 18_B + p124: 10_B
42	p154_{18, 1} : 70_B	70	3	p109: 53_B + p110: 6_B + p111: 11_B:G
43	p156_{18, 3} : 17_B:BB	17	2	p110: 6_B + p111: 11_B:G
44	p158_{18, 5} : 23_B	23	2	p151: 11_B + p152: 12_B
45	p160_{18, 7} : 13_B	13	2	p34: 11_B + p35: 2_B
46	p161_{18, 8} : 37_B	37	2	p113: 16_B + p114: 21_B
47	p163_{19, 1} : 45_B	45	5	p122: 6_B + p123: 18_B + p124: 10_B + p125: 6_B + p126: 5_B
48	p168_{19, 6} : 21_B	21	2	p141: 18_B + p142: 3_B
49	p172_{20, 1} : 28_B	28	2	p123: 18_B + p124: 10_B

Khipu Notes:

Ashok Khosla Notes:
This khipu has been entered with a strange nonconsecutive numbering scheme, indicating either the clusters are off, or the pendants are misnumbered. Investigation into the databook is warranted.

Ascher Databook Notes:

The khipu is attached to a carved wooden bar.
UR1136 and UR1140 were acquired by the Museum in 1904. The provenance is given as Nasca. For a comparison of them, see UR1136.
UR1140 is discussed by Nordenskiold (see Introduction) .A photograph of it appears in Schmidt, Max, 1929, Kunst und Kultur von Peru, Impropyläen-Verlagzu, Berlin.
The way in which the bar is threaded separates the khipu into 2 parts each containing 10 groups of 9 pendants each

Note: The pendant order on the listing proceeds from one end of the bar to the other, goes around the end, and continues on the other side. However, similar color and number patterns are found in both parts if the pendants are read instead from one end of the bar to the other, and then beginning at the original end on the other side of the bar. We will, therefore, refer to the pendants and groups on side 2 as if following the second scenario. Part 1 is pendants 1--->90 and references to the j^th pendant in the i^th group correspond to pendant 9 (i-1) +j. Part 2 is pendants 180--->91 and references to the j^th pendant in the i^th group correspond to pendant 190-9i-j.

By color, each part is separated into 2 subparts of 6 groups and 4 groups respectively. Each group has the same color pattern: 5 B, 2 mixed, 2 B. In the first 6 groups in part 1, the 2 mixed cords are B:BB/B, and in the last 4 groups they are B:BB. In the first 6 groups in part 2, the 2 mixed cords are B:BB, and in the last 4 groups they are B:G.
Subsidiaries are only on the last pendant in each group in part 1. All are BB.
In all groups in both parts:
1. The last pendant in each group has the maximum value.
2. With the exception of group 10 in part 1, the value on pendant 7 is always great than the value on pendant 8.
Within part 1:
1. For the 6 groups of the first subpart, the sum of the values in the 5th position equals the sum of the values in the 4th position. That is:
  
  \[ \sum\limits_{i=1}^{6} P_{i5} = \sum\limits_{i=1}^{6} P_{i6} \]
2. For the 4 groups of the second subpart, the sum of the values in the first position equals the sum of the values in the second position.
  
  \[ \sum\limits_{i=7}^{10} P_{i1} = \sum\limits_{i=7}^{10} P_{i2} \]
3. Excluding the last pendant in each group, the sum of the values in the first group equals the sum of the values in the corresponding group in the second subpart (group 7).
  
  \[ \sum\limits_{j=1}^{8} P_{1j} = \sum\limits_{j=1}^{8} P_{7j} \]