AS026B/KH0028

Original Author: Marcia & Robert Ascher
Museum: University Museum, University of Pennsylvania
Museum Number: SA4030
Provenance: Unknown
Region: Unknown 		Total Number of Cords: 260
Number of Ascher Cord Colors: 9
Similar Khipu:  Previous (AS048)  Next (AS186)
Catalog: AS026B
Khipu Notes: Khipu Notes

Use ⌘ + or ⌘ – to Zoom In or Out

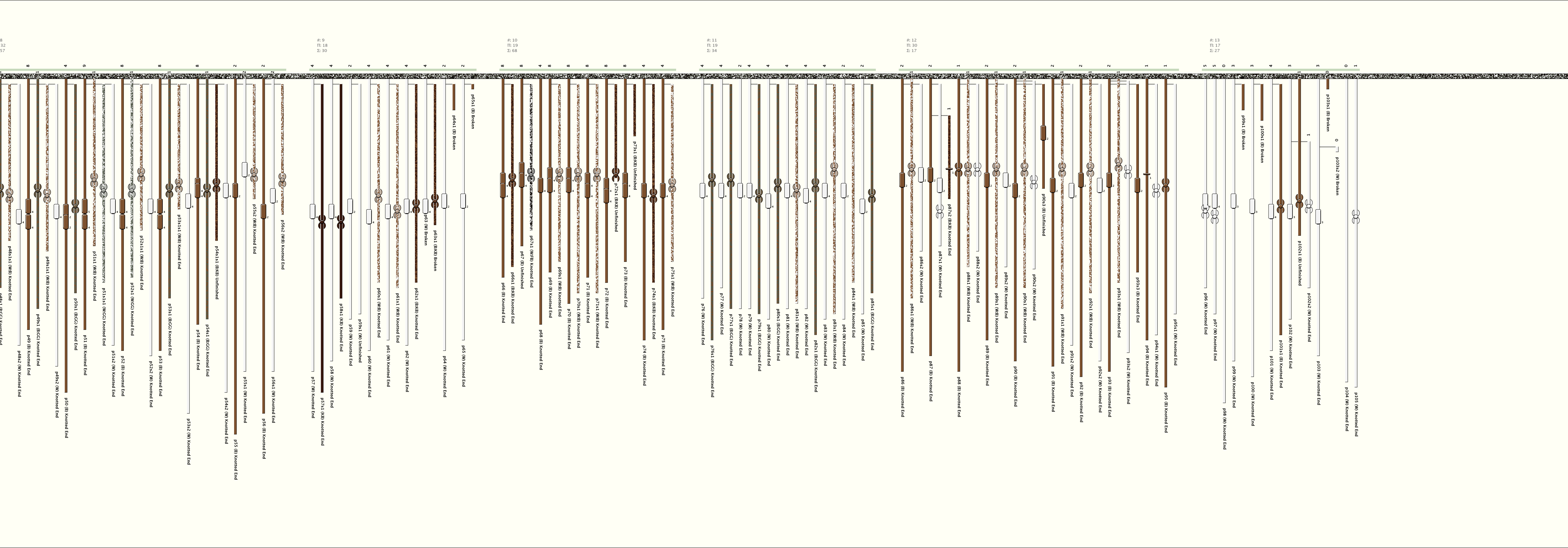
Khipu Notes

Fieldguide Notes:

This khipus has 1 interesting field-mark:
  1. With the exception of groups 3 and 5, each of the 11 groups have a number scheme that gets halved over the pendants. For example Group 1, has (2) 24's, (2) 12's, (5) 24's, and (2) 12's. Each group can be described in terms of one value.
    Calling this value N, basically the pendants in the group contain:
    \[N, N, {N \over 2}, N, N, N, N, N, {N \over 2}, {N \over 2} \]

Ascher Databook Notes:
  1. Khipus AS026A and AS026B were joined. Their linkage and a comparison of their colors are detailed with AS026A.
  2. Most of the subsidiaries (103 of 150) have one knot. While some are single knots and some are figure eight knots, their positions on the cords are the same. Both types have been interpreted as value 1.
  3. By spacing, there are 11 groups and 2 single pendants. The color of the pendants in the first 2 groups is W; in the next 3 groups all are MB; and for groups 6-11, the colors alternate. Group 6 is MB, group 7 is W, group 8 is MB, etc.
    Both single pendants are W.
  4. The groups that have 9 or 10 pendants (with the exception of group 11) have values that are similarly internally related. The relationship is of parti­cular interest because it involves halving.
    These groups, discussed below in greater detail, are groups 1, 2, 4, 6, 7, 8, 9, 10.

    1. Each group can be described in terms of one value. Calling this value N, basically the pendants in the group contain:
      \[N, N, {N \over 2}, N, N, N, N, N, {N \over 2}, {N \over 2} \]
      Where there are 9 rather than 10 pendant positions, one of the 5 consecutive positions with value N is non-existent. The values involved are:
      \[N=24; ({N \over 2}=12); N=9; ({N \over 2}=4); N=5; ({N \over 2}=4); N=8; ({N \over 2}=4); N=4; ({N \over 2}=2); N=8; ({N \over 2}=4); N=4; ({N \over 2}=2); N=2; ({N \over 2}=1)\]
      1. Where N=8 (in groups 4, 6, 8), the value 8 is represented by a long knot of 4 immediately followed by a long knot of 4, rather than by a long knot of 8. Half of it is one long knot of 4 (in groups 4 and 8).
        By con­trast, there is a double long knot of 2 in group 6. It is the distributive axiom, of course, that justifies the use of these 2 procedures to arrive at equivalent results.
        Namely, A = (A+A)/2 = (A/2) + (A/2)
      2. Since halving when only integers are used causes problems for odd values, it is important to note that 9/2 → 4.

    2. Including subsidiary values, the values of the positions change but the basic internal pattern remains the same. Since there are 0-3 subsid­iaries per pendant position, there are more inconsistencies.
      The values and their representations are detailed:

      Group 1:    N=28+24+2+2; N/2=14=12+1+1 (with 1 inconsistency)
      Group 2:    N=11=9+1+1; N/2=5=4+1
      Group 4:    N=9=4+4+1; N/2=5=4+1
      Group 8:    N=9=4+4+1 ; N/2=4 in 1 position, N/2=4+1 in 2 positions
      Group 7:    N=5=4+1; N/2=2 in 1 position, N/2=2+? in 2 positions
      Group 9:    N=5=4+1; N/2=2 in 2 positions, N/2=2+1 in 1 positions
      Group 10:   The values of N vary (4 are N=5=2+2+1 and 3 are N=6=2+2+1+1). Also the N/2 position values vary (one is 1, another is 1+1, and one is 1+1+1).
      Group 6:    N=14=4+4+4+1+1 ; N/2=5=2+2+1 (Note: In each of the 3 representations of N/2, one of the 4's in N has been ignored. This might be viewed as N=10; N/2 = 5)

      1. Comparison of the arithmetic in groups 1 and 2 again shows the two different orders of computation: 28/2=14 uses (A+B+B)/2 = A/2 + B/2 + B/2 while 11/2 = uses (A+B+B)/2 = A/2+ 2B/2 = A/2 + B
      2. The odd numbers present are 11, 9, 5. Note that 11/2 = 5 in all positions and 5/2=2 in 3 of 6 positions. However, 5/2=3 in one position and is unclear (2+? ) in 2 positions.
        Also, 9/2 now equals 5 in 5 of 6 positions. It might be that the representation of 9 by 4+4+1 is leading to this alter­nate result.
        Overall, in halving 18 odd numbers, 10 of them are rounded down, 6 are rounded up, and 2 involve broken cords.
    3. The focus on halving is also seen by comparing the basic values of consecutive groups. The values of N (without subsidiaries) for groups 6-10 are 8, 4, 8, 4, 2.
      Within each group, the number of consecutive pendants with repeated values also shows a halving pattern: two have value N followed by 1 of value N/2; then 4 or 5 have value N followed by 2 of value N/2.